Editor’s note: Do you tackle a Sudoku on your cottage veranda, sailboat cockpit, or at a campsite? TI Life is taking full advantage of Dan LeKander, from Wellesley Island, who is a sudoku expert and author of “3 Advanced Sudoku Techniques – That Will Change Your Game Forever!” This is Part 2 of 6 parts of his 8 step approach.
|
After completing the puzzle preparation (TI LIFE August issue link), by filling-in all obvious answers and indicating the options at the top of the unsolved cells, you are ready to use techniques to arrive at a solution to the Sudoku Puzzle.
This article explains Step 1, which involves identifying Pairs, Triplets and Quads, to reduce the number of options in the unsolved cells. Finding Pairs, Triplets and Quads is like a game, within a game, and the challenge can be fun!
PAIRS … To explain this technique it is best to look at actual examples:
Example 1a
|
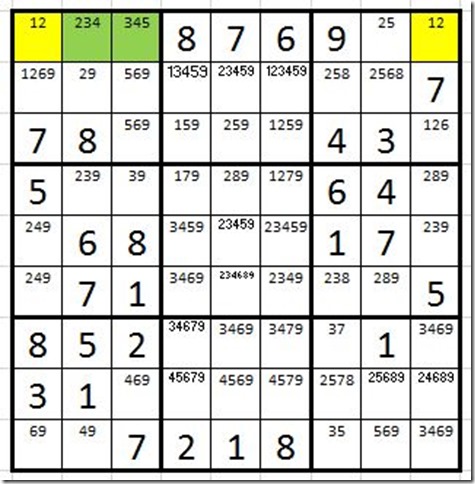
In Example 1a you find two cells in row 1 (highlighted in yellow), which have two options: 1 and 2. We will call this an obvious pair; what does that mean?
The pair is easily identified, in that there are two cells in row 1, which have the same two options, and no other options. Therefore, with regard to row 1, a 1 or 2 cannot exist in any other cells in row one, therefore cell C8R1 (column 8, row 1) = 5.
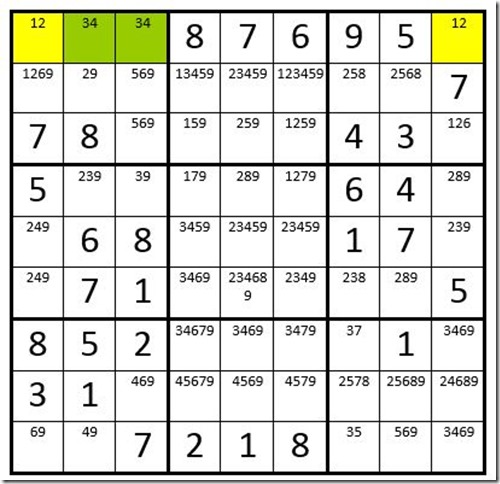
Row 1 actually has another pair, highlighted in green; this is called a hidden pair. C2R1 and C3R1 are the only two cells in row 1, which contain the two options, 3 and 4. Therefore, all other options for those two cells can be eliminated and your grid looks like example 1b below.
Example 1b
|
Example 1b has another hidden pair; can you find it? See “ANSWERS” near the end of this article
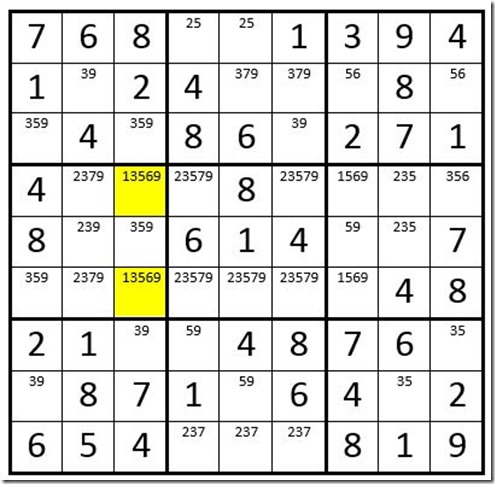
Example 2a below shows another hidden pair. The two yellow highlighted cells are the only cells, in column 3, which contain the two options 1 and 6. Therefore all other options for those two cells can be eliminated.
Example 2a
|
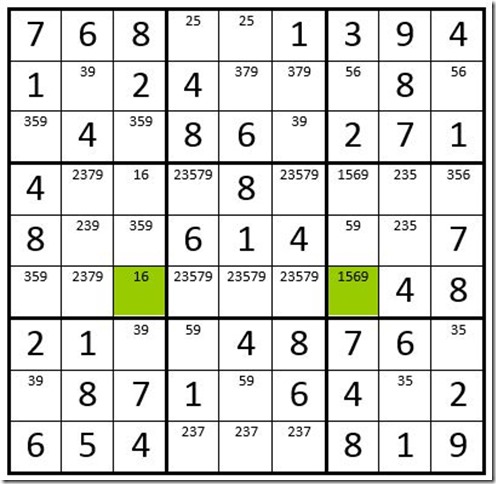
Now your grid should look like Example 2b below.
Example 2b
|
Notice that the two green highlighted cells in row 6, are the only two cells in row 6, which have 1 and 6 as options. Therefore the 5 and 9 in C7R6 can be removed as options.
Pairs can exist in Rows, Columns, and Boxes. To detect pairs, search each row, then column, and then box.
TRIPLET … Example 3 below, is an example of an obvious triplet.
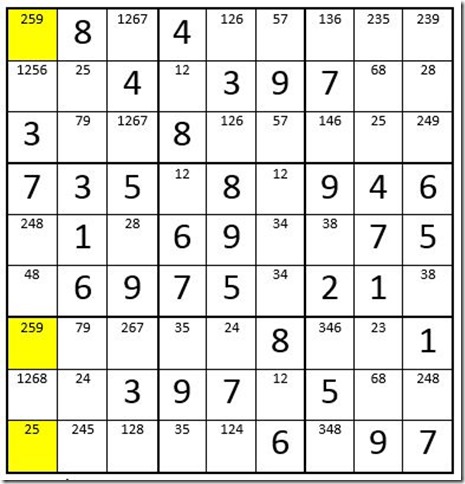
Example 3
|
In Example 3, you find that the three yellow highlighted cells, in column 1, contain the three options 2, 5 and 9. Therefore, just like pairs, this “triplet” has a monopoly, in column 1, for the numbers 2, 5 and 9, and these numbers cannot exist, in column 1, for any other unsolved cells. You may now remove the 2 and 5 from C1R2, the 2 from C1R5, and the 2 from C1R8.
Example 3 has another obvious triplet. Can you find it?
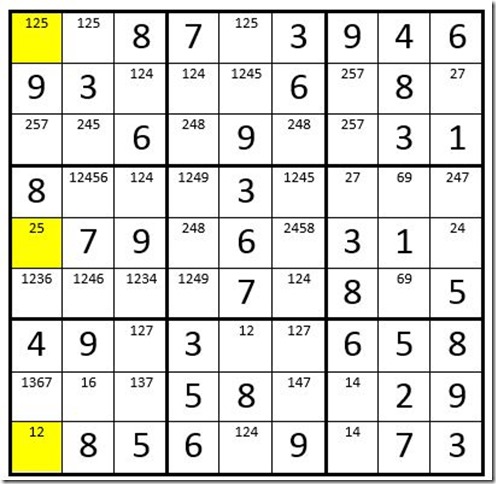
Example 4 below has another obvious triplet, with the three cells highlighted in yellow. You can remove the 1, 2 and 5, from the other cells in column 1.
Example 4
|
Example 5a below, is an example of a hidden triplet, which is much more difficult to detect than an obvious triplet. You may note that the three options, 4, 5 and 7, are contained in only the three yellow highlighted cells, in row 7, and therefore the other options in those three (yellow highlighted) cells, may be eliminated.
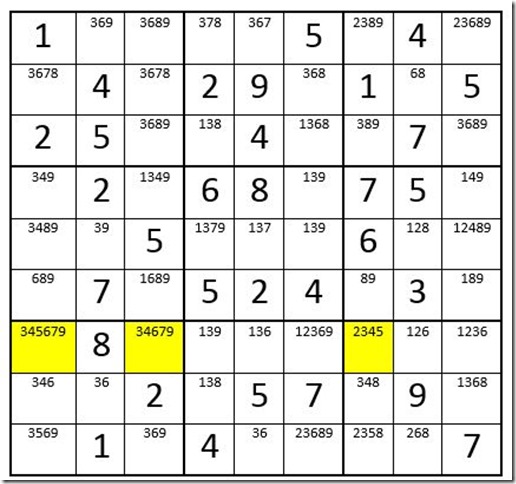
Example 5a
|
Now your grid should look like this Example 5b below.
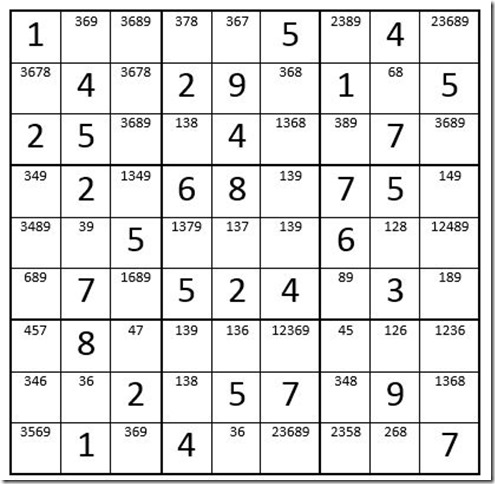
Example 5b
|
Triplets can exist in Rows, Columns, and Boxes. To detect triplets, search each row, then each column, and then each box.
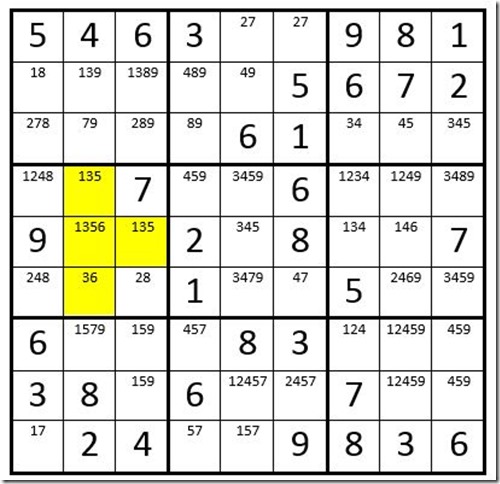
QUADS … Example 6 below, is an example of an obvious quad.
Example 6
|
In Box 4, the four yellow highlighted cells, exclusively contain the four options, 1, 3, 5 and 6. Therefore the 1 can be eliminated from C1R4. (You can also look at this in another way. The cells in box 4, which are not highlighted, are actually a hidden triplet. C1R4, C1R6 and C3R6 are the only three cells, which contain the three numbers, 2, 4 and 8. Therefore the 1 can be eliminated from cell C1R4.)
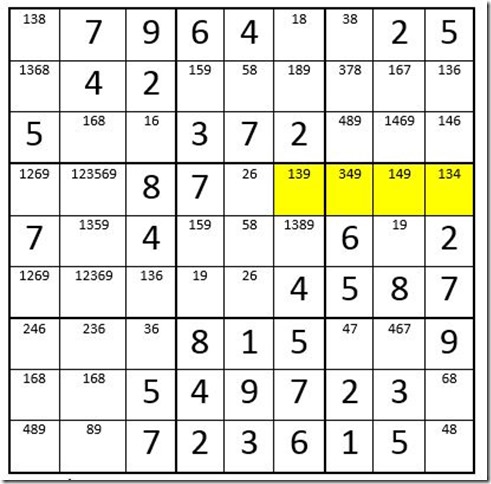
Example 7
|
Example 7 is another obvious quad. In Row 4, you find that four yellow highlighted cells, which contain only the four options, 1, 3, 4 and 9. Therefore the 1, 3, 4, and 9 can be removed from the other cells in row 4 (again, the other unsolved cells in row 4 form a hidden triplet 2, 5, 6).
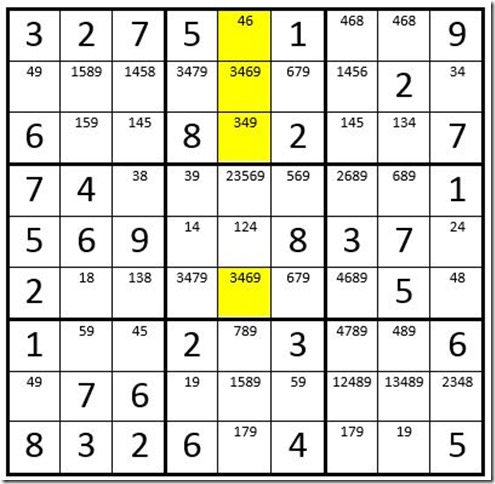
Example 8
|
Example 8 is another obvious quad. In Column 5, the four yellow highlighted cells, contain only the four options 3, 4, 6, and 9. Therefore these numbers may be removed from the other cells in column 5.
The Grid in Example 8 also contains a hidden triplet; can you find it?
Quads can exist in Rows, Columns, and Boxes. To detect quads, search each row, then column, and then box.
Conclusion
Obvious Pairs, Triplets, and Quads are relatively easy to detect. Hidden Pairs, Triplets, and Quads are sometimes not so easy. As you gain experience, with these techniques, you will more easily detect these patterns, and as you scan each row, column, and box, you will be able to look for pairs, triplets, and quads, at the same time. Obviously, this technique has great rewards and can drastically reduce the time to finish a Sudoku Puzzle.
Answers
- Example 1b hidden pair … C4R4 and C6R4 contain the pair 1, 7.
- Example 3 obvious triplet … C1R9, C2R8, and C2R9 contain the triplet 2, 4, 5. Therefore, the numbers 2, 4, and 5cannot exist in the other unsolved cells, in box 7.
- Example 8 hidden triplet … C2R2, C3R2, and C7R2 contain the triplet 1, 5, 8.
|
Conclusion of Part 2, of a 6 Part Series: The next article will cover the techniques: “Interaction” and “Turbos”.
For those readers who wish to purchased Dan’s book, it is available at two locations online, (djlsuniverse.com, amazon.com and ebay.com).
Purchase of a book includes a 50 page blank grid pad, 33 black and two green tokens, to assist with Step 6.…
|
By Dan LeKander, Wellesley Island, NY
Dan LeKander and his wife, Peggy, have been seasonal residents of Fineview on Wellesley Island since 1983. In addition to being a Sudoku addict, Dan explores the 1000 Islands to enjoy the wildlife, beauty, and of course, Catch-and Release bass fishing.
[See Jessy Kahn’s Book Review, “3 Advanced Sudoku Techniques…” by Dan LeKander, June issue of TI Life.
]