A Complete Guide to Solving a Difficult Puzzle – November 2016
What are your Sudoku aspirations? Do you want to pass time in an airport? Do you want to be able to solve some difficult puzzles? Is it worth your time to become a Sudoku master and be able to solve any Sudoku puzzle? For this article and articles in the months to follow, this new format should allow you to take this passion to any level you desire!
First, we will revisit the approach as discussed in Dan’s first six TI LIFE articles …
| |
DAN’S 8 STEP APPROACH TO SOLVING ALL SUDOKU PUZZLES
Once you have completed the puzzle to the extent that you have filled in all obvious answers and have written all potential options across the top of the unsolved cells (PUZZLE PREPARATION), Dan recommends the following steps to complete the puzzle.
See TI Life Puzzle Preparation:
Step 1: Sudoku Pairs, Triplets and Quads – September, 2015
Step 2: Turbos & Interaction – October, 2015
Step 3: Sudoku Gordonian Rectangles and Polygons – November, 2015
Step 4: XY-Wings & XYZ Wings – December, 2015
Step 5: X-Wings – January, 2016
________________
Step 6: DAN’S YES/NO CHALLENGE
Step 7: DAN’S CLOSE RELATIONSHIP CHALLENGE
Step 8: AN EXPANSION OF STEP 7
Steps 1-5 are relatively common techniques and are explained in the TI LIFE articles per above. Steps 6-8 are covered in detail, in Dan’s book.
Also see Sudoku Puzzle Challenge… February 2016, Sudoku Puzzle Challenge–March 2016, Sudoku Puzzle Challenge–April 2016,May 2016, June 2016, July 2016, August 2016, September 2016 and October 2016.
|
|
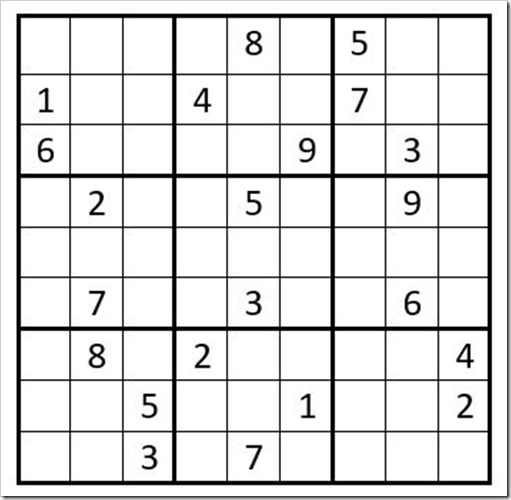
Puzzle #19
|
You have a number of choices as to how to approach Puzzle #19. You can print it and give it a go. If you need help, you can visit the step-by-step solution explained below. If you are good at Puzzle Preparation, you can compare your results with 19.5 below. If you are a beginner, you can witness all the steps of solving this puzzle. Regardless of your level of expertise or your approach, you can learn as much as you please from this puzzle.
Puzzle Solution
Let’s keep in mind that a number cannot repeat itself in a row, column, or box. Also, there can only be one solution to the puzzle (which is the basis for Step 3: Gordonian).
Puzzle Preparation
OBVIOUS ANSWERS … There is only one cell in box 7 (lower left) that can contain a 2, which is C1R9 (cell in column 1, row 9), as shown in 19.1 below.
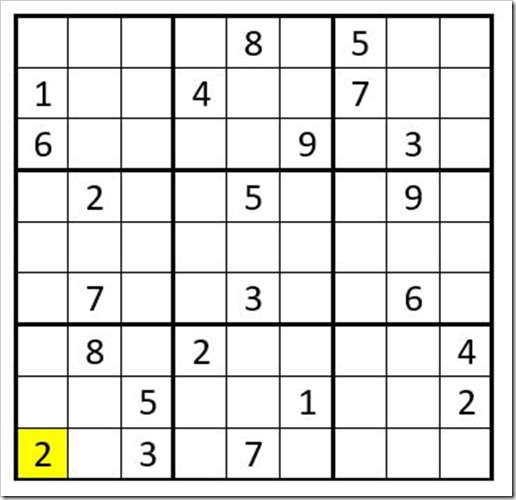
Puzzle #19.1
|
NUMBERS IN UNSOLVED CELLS THAT CAN NOT EXIST… Next we will explore numbers (options) that cannot exist in particular unsolved cells. First, we will look at the 3’s in 19.1. In box nine (lower right) a 3 can only exist as an option in C7R7 or C7R8. Therefore a 3 cannot exist in C7R4, C7R5 or C7R6 and we will mark it as such in 19.2 below. So in the future if an option cannot exist in a cell we will put that in the bottom of the cell, and later we will put the options that can exist in a cell in the top of the cell.
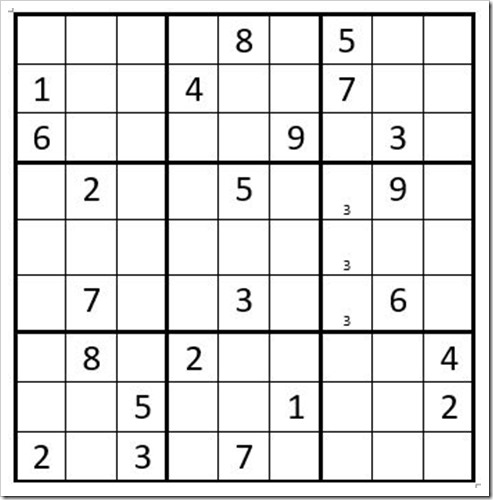
Puzzle #19.2
|
If we look at 19.2 above and explore the 5’s, 6’s, 7’s & 8’s we find similar situations, resulting in 19.3 below

Puzzle #19.3
|
FILL IN THE OPTIONS FOR THE UNSOLVED CELLS
Usually I start with the row, column or box which has the most solved cells, and in this example it would be column five with 4 solved cells. You can see that the options for C5R2 are 2 & 6. We will mark “26” in C5R2 as per 19.4 below.
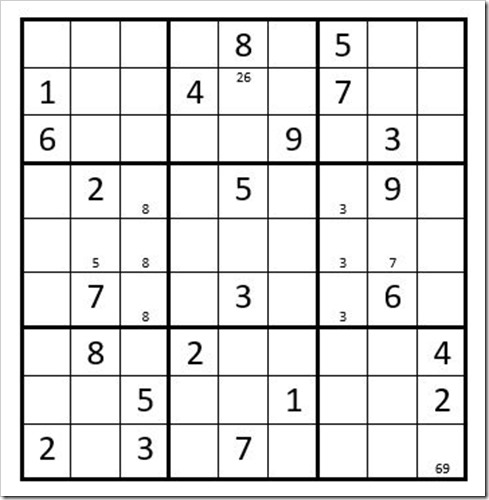
Puzzle #19.4
|
Next I would fill in the options for the rest of column 5, then continue until all unsolved cells have been filled in. However, jumping ahead we will look at the possible options for C9R9. Normally the options would be 1,5,6,8,9, but we have said that that cell cannot be a 6 or 9. Therefore the options for that cell are 158. When you fill in C9R9, you can erase the 6 and 9.
Now fill in the options for the remaining unsolved cells. When you finish filling in the options, your grid should look like 19.5 below.
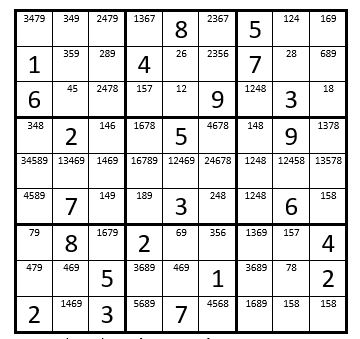
Puzzle #19.5 Through Puzzle Preparation
|
On the surface this puzzle looks rather difficult! Is it difficult or is difficulty an illusion? What is next? Yes, Steps 1-8!
STEPS 1-8
STEP 1: PAIRS, TRIPLETS & QUADS … In row two we find an obvious quad of 2689 with cells C3R2, C5R2, C8R2 & C9R2, eliminating the 9 from C2R2 and the 2 & 6 from C6R2. (Or you could look at row two in a different perspective … C2R2 & C6R2 are the only two cells in row two that contain the options 3 & 5. Therefore those two cells will have options 35, and you can eliminate the other options in those two cells. (This is called a hidden pair.) Now the grids looks like 19.6 below.

Puzzle #19.6
|
STEP 1: PAIRS, TRIPLETS & QUADS … In column seven we find an obvious quad 1248 in cells C7R3, C7R4, C7R5 & C7R6. The 1248 can be removed from the other unsolved cells in column seven, and now the grid looks like 19.7 below.
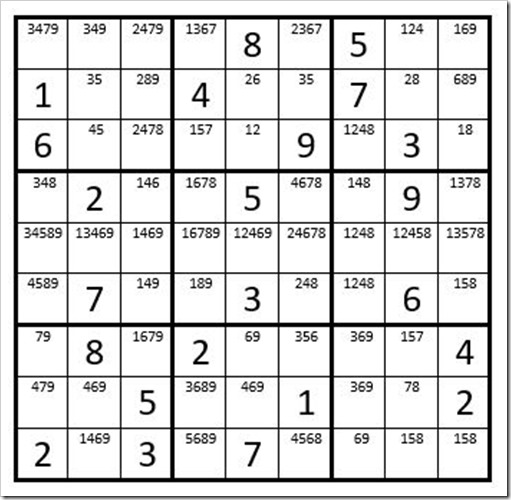
Puzzle #19.7
|
STEP 1: PAIRS, TRIPLETS & QUADS … In column nine we find that the only two cells that can contain a 6 & 9 are C9R1 & C9R2 (hidden pair). Therefore, we can eliminate the other options from these two cells, giving us 19.8 below. (You could also have looked at this column and spotted an obvious quint, 13578, eliminating any of those options from C9R1 & C9R2).
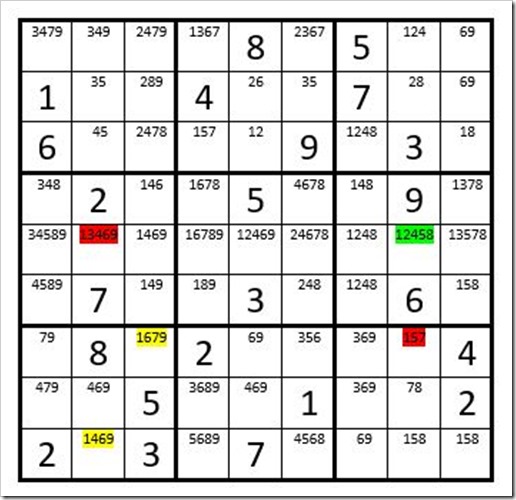
Puzzle #19.8
|
Step 2: INTERACTION & TURBOS … In 19.8 above when searching for Interactions & Turbos you find only two unsolved cells in box seven that contain 1, as an option that are neither in the same row or column (highlighted in yellow), setting the stage for a Turbo.
Go vertical on one column from one of these two cells to find only one other cell in that column to contain a 1, and go horizontal on the row from the other cell to find only one other cell in that row to contain a 1. Those cells are highlighted in red. Where the two red cells intersect is C8R5 highlighted in green. This green cell cannot contain a 1 as an option, resulting in 19.9 below. (Theory refreshment … one of the yellow cells is a 1. If C2R9 is a 1, then C3R7 is not a 1, then C8R7 is a 1, and C8R5 is not a 1. If C3R7 is a 1, then C2R9 is not a 1, then C2R5 is a 1, then C8R5 is not a 1.
So, regardless which yellow cell is a 1, C8R5 is not a 1, eliminating the 1 from that cell.) Turbos are a lot of fun! Best of all, it works every time! Once you understand the theory you just perform these simple steps to eliminate an option in a cell.
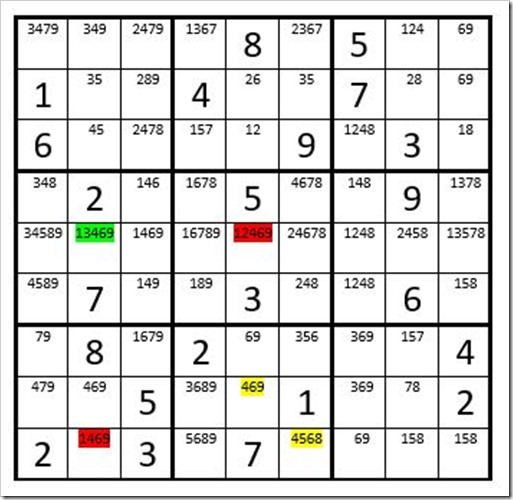
Puzzle #19.9
|
Step 2: INTERACTIONS & TURBOS … In 19.9 above, in box 8 (lower center) we find another start of another Turbo. This time you can eliminate the 4 from C2R5, resulting in 19.10 below.
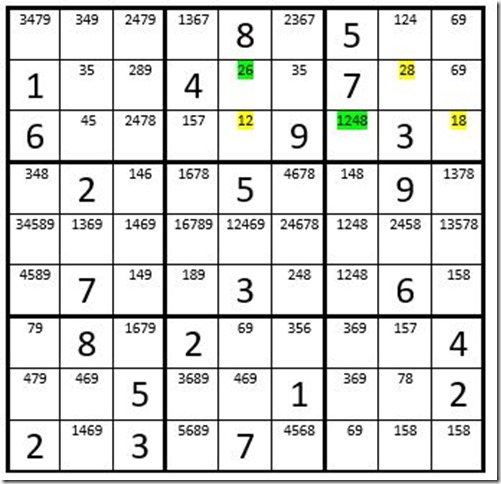
Puzzle #19.10
|
Step 4: XY-WINGS & XYZ WINGS … In looking at box 3 (upper right) in 19.10 above we see one cell with options 28 and another cell with options 18. Are there any cells adjacent to either of these two cells that has 12 as an option? Yes, C5R3. The 3 cells that form an XY-Wing are highlighted in yellow.
Which cell in box 3 (upper right) is adjacent (in the same row or column) to the other two yellow highlighted cells? Yes, C9R3, so it becomes the “driver cell”. Since the theory is rather straightforward, here we go! If C9R3 is a 1, then C5R3 is a 2, which would prevent C5R2 and C7R3 (highlighted in green) from being a 2. If C9R3 is an 8, then C8R2 is a 2, which would prevent C5R2 and C7R3 (highlighted in green) from being a 2.
Since C9R3 has to be a 1 or 8, in neither case can the green cells be a 2. Therefore the 2 can be eliminated from the two green cells as an option, resulting in 19.11 below.
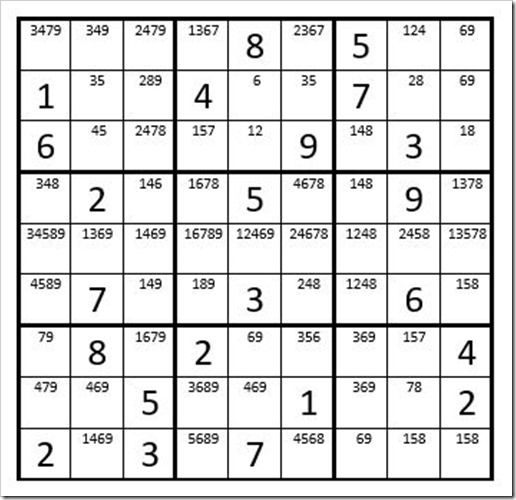
Puzzle #19.11
|
Eureka, that leaves a 6 as the only option for C5R2, so we will mark it a 6,
- then C9R2=9
- C9R1=6
- C5R7=9
- C5R8=4
- C1R7=7, and so forth
The puzzle is disarmed and easily surrenders from here. X-Wings, XY-Wing & XYZ-Wings are a barrel of fun! With a little practice and an understanding of the theory, your Sudoku techniques can be greatly enhanced.
Editor’s note:
Do you tackle a Sudoku on your cottage veranda, sailboat cockpit, or at a campsite? TI Life is taking full advantage of Dan LeKander, from Wellesley Island, who is a Sudoku expert and author of “3 Advanced Sudoku Techniques – That Will Change Your Game Forever!”
In January 2016, we published a final article in his series – but many of us enjoy using “Dan’s Steps,” so when he asked if we would like a puzzle to solve every month … this editor said an enthusiastic… Yes, please!
I suggest you try this relatively easy puzzle and that you also purchase Dan’s book, “3 Advanced Sudoku Techniques, That Will Change Your Game Forever!”
Most importantly, I ask that you leave comments on any part of his series and throughout the year. Remember when your teacher said – no such thing as a silly question – as we can all learn together.
Dan’s book is available online, djlsuniverse.com, amazon.com and on ebay.com.
Purchase of a book includes a 50-page blank grid pad, 33 black and two green tokens, to assist with Step 6.…
I want to thank Dan… what a lot of work he puts into our TI Life articles. And another thank you goes to Bill Stallan, who proofreads the content each month!)
|
Do you have any suggestions for future articles? You can post on this TI Life issue, or contact me directly at my e-mail address … djlsuniverse@yahoo.com.
Thank you for your interest. May the gentle winds of Sudoku be at your back!
Dan LeKander