Another complete guide to solving a difficult puzzle, December 2016.
Puzzle #20 has much to offer in terms of learning and practicing common & advanced Sudoku techniques. A step-by-step guide is provided for you to follow the process if you need assistance.
First, we will revisit the approach as discussed in Dan’s first six TI LIFE articles …
| | DAN’S 8 STEP APPROACH TO SOLVING ALL SUDOKU PUZZLES Once you have completed the puzzle to the extent that you have filled in all obvious answers and have written all potential options across the top of the unsolved cells (PUZZLE PREPARATION), Dan recommends the following steps to complete the puzzle. See TI Life Puzzle Preparation: Step 1: Sudoku Pairs, Triplets and Quads – September, 2015 Step 2: Turbos & Interaction – October, 2015 Step 3: Sudoku Gordonian Rectangles and Polygons – November, 2015 Step 4: XY-Wings & XYZ Wings – December, 2015 Step 5: X-Wings – January, 2016 ________________ Step 6: DAN’S YES/NO CHALLENGE Step 7: DAN’S CLOSE RELATIONSHIP CHALLENGE Step 8: AN EXPANSION OF STEP 7 Steps 1-5 are relatively common techniques and are explained in the TI LIFE articles per above. Steps 6-8 are covered in detail, in Dan’s book. Also see Sudoku Puzzle Challenge… February 2016, Sudoku Puzzle Challenge–March 2016, Sudoku Puzzle Challenge–April 2016, May 2016, June 2016, July 2016, August 2016, September 2016, October 2016 and November 2016 |
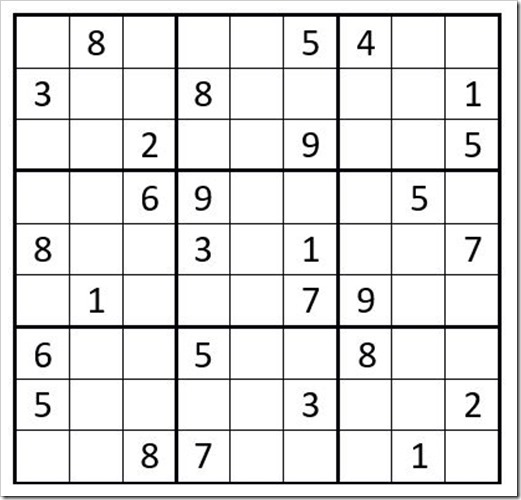
Puzzle #20 |
Decision time – do you want to print Puzzle #20 and see if you can solve it, prior to following the steps provided below? Or do you want to stroll through the puzzle with me as I utilize Dan's 8 Step Approach in solving this puzzle?
PUZZLE SOLUTION
First of all, let’s keep in mind that a number cannot repeat itself in a row, column or box. Also, there can only be one solution to the puzzle.
PUZZLE PREPARATION
OBVIOUS ANSWERS … Starting with the 1’s, there is only one cell in box 6 (middle right) that can contain a 1, which is C7R4 (cell in column 7, row 4), as shown in 20.1 below.
| 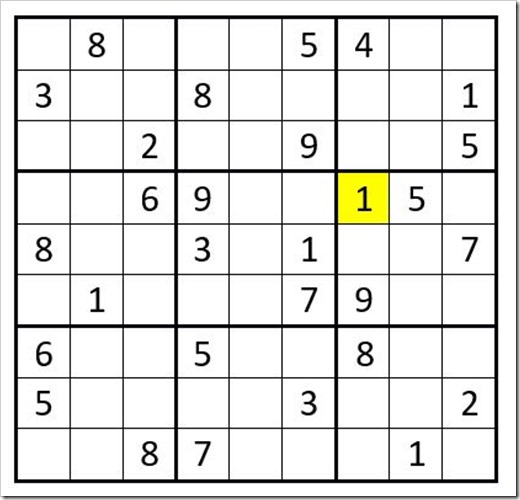
Puzzle #20.1 |
Without looking at 20.2 below that can you find the rest of the obvious answers which also exist in 20.1 above?
- In box 9 (lower right) a 5 can only exist in C7R9
- In box 3 an 8 can only exist in C8R3
- In box 8 an 8 can only exist in C5R8
- In box 5 an 8 can only exist in C6R4
- In box 6 an 8 can only exist in C9R6
The grid should look like 20.2 below …
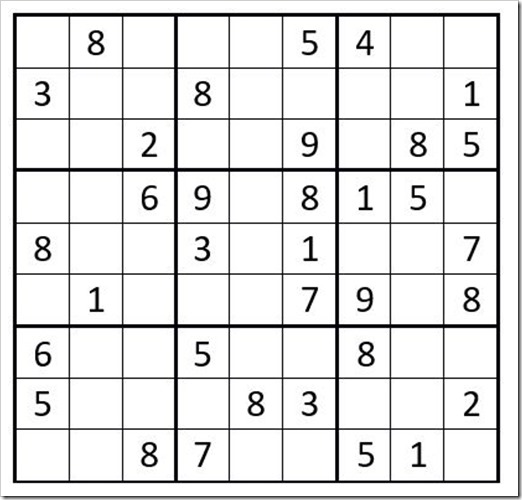
Puzzle #20.2 |
NUMBERS IN UNSOLVED CELLS THAT CANNOT EXIST … Next we will explore numbers (options) that cannot exist in particular unsolved cells. First, we will look at the 1s. In box 7, (lower left) a 1 can only exist as an option in C3R7 or C3R8, (because of the 1 in C2R6 and the 1 in C8R9). Therefore a 1 cannot exist in C3R1 or C3R2. We already know that a 1 cannot exist in C3R2, so we will mark C3R1 with a “1” in the lower part of the cell as per 20.3 below. Later, we will put the options that can exist in a cell in the top of the cell. (If you miss this clue, you will find it later when checking for Step 2: Interactions & Turbos.
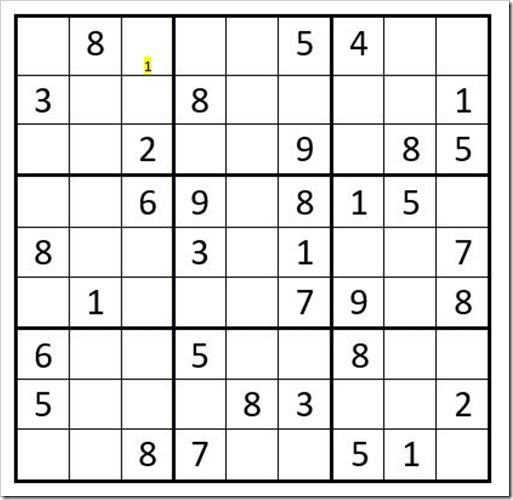
Puzzle #20.3 |
FILL IN THE OPTIONS FOR THE UNSOLVED CELLS
We will start with the row, column or box, which has the most solved cells, and in this example it would be column 6 with 6 solved cells. The options for this column are indicated in 20.4 below.
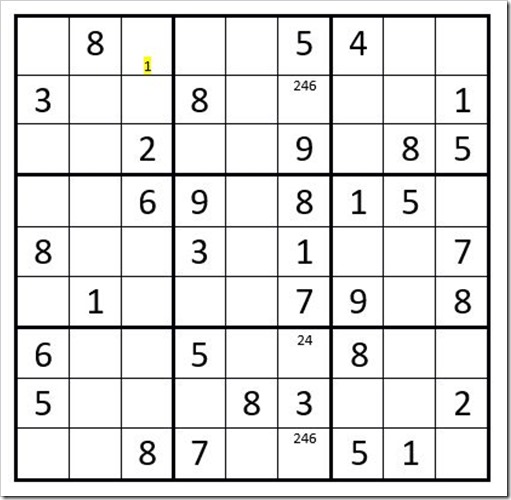
Puzzle #20.4 |
Now continue until all unsolved cells have been filled in. When you fill in the options for column 7, you will find that the only cell that can contain a 3 is R7R3. Then the only cell in box 2 that can be a 3, is C5R1, so indicate a 3 in those two cells. When you finish filling in the options, the grid should look like 20.5 below.
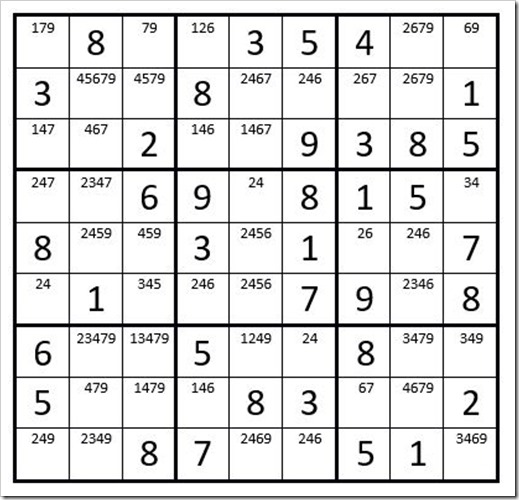
Puzzle #20.5 Through Puzzle Preparation |
STEPS 1-8
STEP 2: INTERACTION & TURBOS … In 20.6 below, when searching for Interactions & Turbos, you find only two unsolved cells in box 6 , which contain 3 as an option that are neither in the same row or column (highlighted in yellow), setting the stage for a Turbo. Go vertical, up or down, on one column, from one of these two cells, to find only one other cell in that column that contains a 3; go horizontal, left or right, on the row from the other cell, to find only one other cell in that row to contain a 3. Those cells are highlighted in red. Where the two red cells intersect is C2R7 is highlighted in green. This green cell cannot contain a 3 as an option. (Theory refreshment … one of the yellow cells is a 3; if C9R4 is a 3, then C8R6 is not a 3; then C8R7 is a 3, and C2R7 is not a 3. If C8R6 is a 3, then C9R4 is not a 3; then C2R4 is a 3, and C2R7 is not a 3. So regardless of which yellow cell is a 3, C2R7 is not a 3, eliminating the 3 from that cell.)
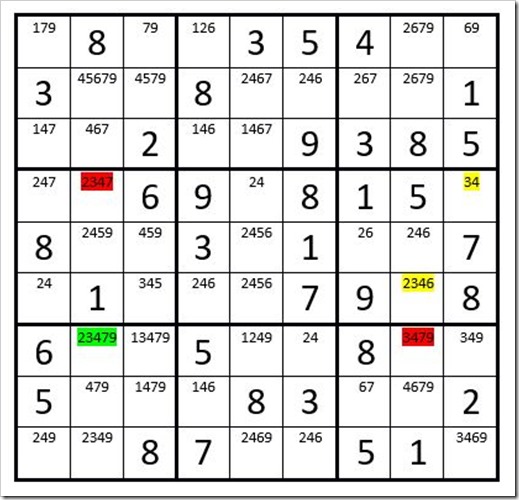
Puzzle #20.6 |
Looking at 20.6 above, there is another Turbo situation in box 4 with 3’s. Can you find the cell that cannot be a 3? (If you need help, follow C2R4 to the right, to C9R4. Follow C3R6 down to C3R7. Where C9R4 and C3R7 intersect is C9R7, which cannot be a 3.
Once you have eliminated the 3’s from those two cells, your grid should look like 20.7 below.
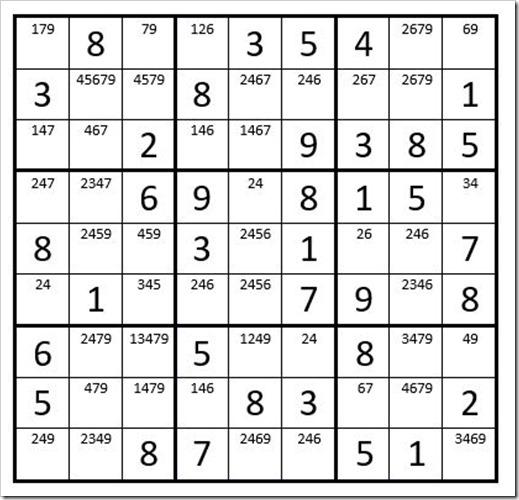
Puzzle #20.7 |
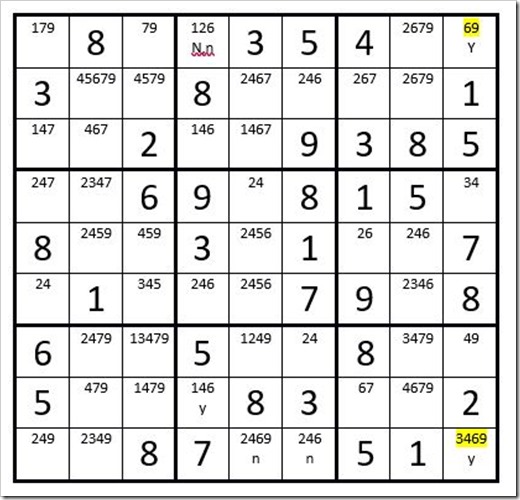
STEP 6: DAN’S YES-NO CHALLENGE … In 20.8 below, when you check for potential step 6 situations, you find two 6’s, as options in column 9. Those cells are highlighted in yellow.
Puzzle #20.8 |
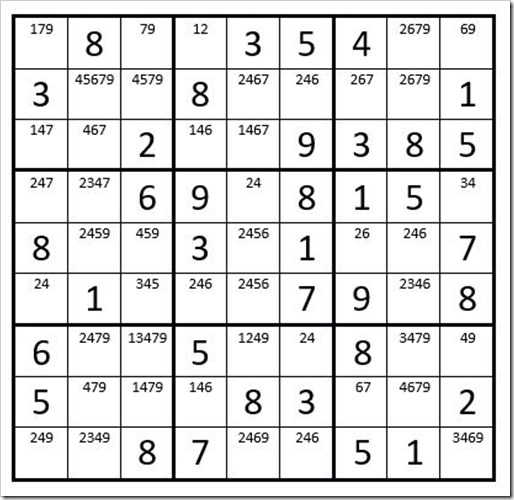
One of the two highlighted cells in column nine, must be a 6. If C9R1 is a 6, then C4R1 is not a 6. We will indicate this by putting a “Y” (for yes) in C9R1 and a “N” (for no) in C4R1. Next, we will assume C9R9 is a 6, and indicate so by putting a “y” (for yes) in that cell. Then C5R9 & C6R9 are not a 6, and we will indicate so by placing an “n” (for no) in those two cells. Now look at box 8 (center lower); if C5R9 & C6R9 are not a 6, then C4R8 must be a 6, so we will indicate a “y” in that cell. If C4R8 is a 6, then C4R1 is not a 6, so we will indicate a “n” in C4R1. Now focus on C4R1; it has a “N,n” designation. Therefore regardless of which cell in column nine is a 6, C4R1 is not a 6, eliminating the 6 as an option from that cell. Your grid should now look like 20.9 below.
Since this was a relatively simple Step 6, you could have mentally figured this out, versus the yes’s and no’s. We did the “yes” and “no” to illustrate the technique. Some Step 6 situations are far more complex and using this technique can eliminate confusion. But when you find a “N,n” you can eliminate the option from that cell with confidence. In my book an example is illustrated where the option in question was eliminated in six cells (during that exercise six cells were marked “N,n” by one Step 6 exercise).
(The relatively simple Step 6 situation above is also known as a “Finned X-Wing”. Without using the technique of Step 6, a Finned X-Wing is sometimes very difficult to detect in a puzzle.)
Puzzle #20.9 |
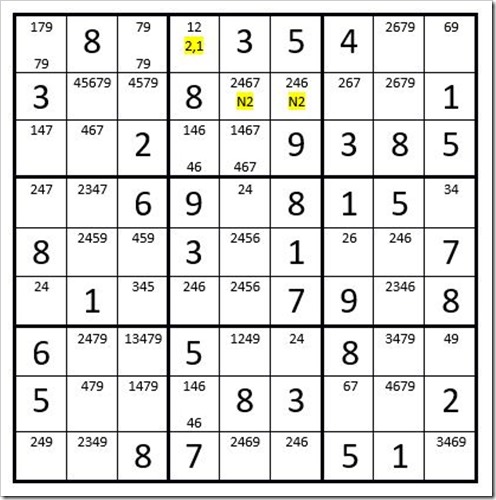
STEP 7: DAN’S CLOSE RELATIONSHIP CHALLENGE … in 20.9 above, we choose any unsolved cell with 2 options, for a Step 7 exercise. We select C4R1, with options 1 & 2. We select the sequence 2,1, as per 20.10 below, and mark C4R1 with “2,1” in the second level of that cell. Theory refreshment … if C4R1 is a 2, then C5R2 & C6R2 cannot be a 2. We will mark those two cells as “N2” in the second level of the cells. If C4R1 is a 1, then only one of cells C5R2 & C6R2 can be a 2 (one of these two cells is an “imposter”). If C4R1 is a 1, then which of cells C5R2 or C6R2 is not a 2? Once we discover this, we can eliminate a 2 as an option from that cell. To start this exercise your grid should look like 20.10 below.

Puzzle #20.10 |
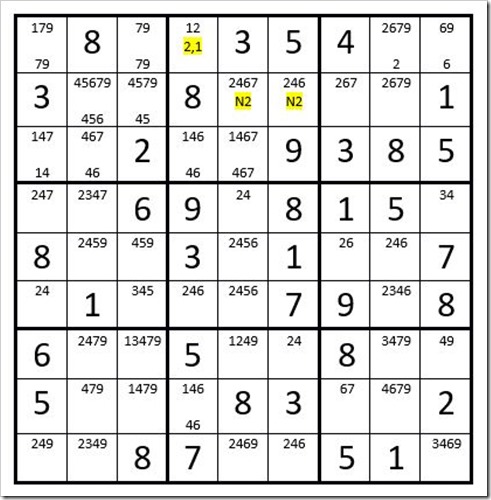
Now track the “1” in cell C4R1 through the puzzle. First, this eliminates the 1 in C1R1, C4R3, C5R3 & C4R8. Your grid should now look like 20.11 below.
Puzzle #20.11 |
You will note that in 20.11 above, we are tracking the 1, by putting the remaining options for the unsolved cells, in the third level of each cell, which becomes a “working level”. By doing this we will not be tampering with the true remaining options, for the unsolved cells in “level 1”. You will also note that C1R1 & C3R1 now form a pair, 79. Then C9R1=6 & C8R1=2. Also, the 7 & 9 can be eliminated as options, for the other unsolved cells in box 1. Your grid should now look like 20.12 below.
Puzzle #20.12 |
Then C7R2=7 & C8R2=9. Continue this track and your grid should look like 20.13 below.
| 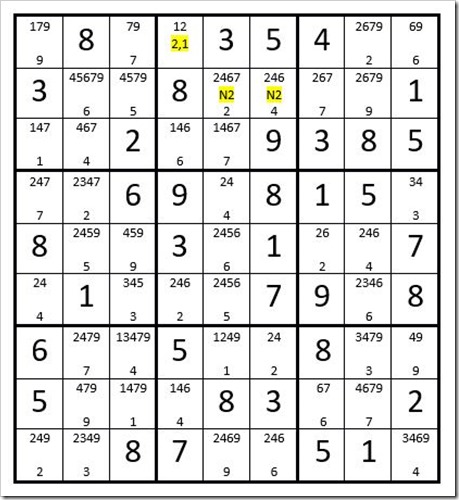
Puzzle #20.13 |
Ok, what did we learn from this exercise? First we learned that C6R2 is a 4,, if C4R1 is a 1. So regardless if C4R1 is a 1 or 2, C6R2 is not a 2, eliminating a 2 as an option for that cell. Secondly, we were lucky that the 1 tracked entirely through the puzzle. Performing a quick check of the 3rd level of each unsolved cell, to see if a number was repeated in a column, row or box, we find that a number was not repeated. So we have a bonus of successfully completing the puzzle. (Had we discovered a repeated number in a column, row, or box, then we could have concluded that C4R1 is not a 1, and is therefore a 2.)
Not all 2nd digits of a Step 7, track through to completion. If it just tracks though to the extent it uncovers the “imposter(s)” in the yellow cells, it is still a successful Step 7! It is accurate to say that not all Step 7 exercises will be successful, or highly productive. In 95+% of difficult Sudoku puzzles you will find at least one Step 7 that is successful. From the results of that success, another Step 7 will generally be successful, and so on. Please also note that sometimes multiple Step 7’s are required to solve a really difficult puzzle.
Step 7: Dan's Close Relationship Challenge is an extremely powerful technique. Mastering this technique will be your most effective means of solving complex Sudoku puzzles.
Thank you for your interest. May the gentle winds of Sudoku be at your back!
Editor’s note: Do you tackle a Sudoku on your cottage veranda, sailboat cockpit, or at a campsite? TI Life is taking full advantage of Dan LeKander, from Wellesley Island, who is a Sudoku expert and author of “3 Advanced Sudoku Techniques – That Will Change Your Game Forever!” In January 2016, we published a final article in his series – but many of us enjoy using “Dan’s Steps,” so when he asked if we would like a puzzle to solve every month … this editor said an enthusiastic… Yes, please! I suggest you try this relatively easy puzzle and that you also purchase Dan’s book, “3 Advanced Sudoku Techniques, That Will Change Your Game Forever!” Most importantly, I ask that you leave comments on any part of his series and throughout the year. Remember when your teacher said – no such thing as a silly question – as we can all learn together. Dan’s book is available online, amazon.com and on ebay.com. Purchase of a book includes a 50-page blank grid pad, 33 black and two green tokens, to assist with Step 6.… I want to thank Dan… what a lot of work he puts into our TI Life articles. And another thank you goes to Bill Stallan, who proofreads the content each month!) |
Dan LeKander, Wellesley Island
Do you have any suggestions for future articles? You can post on this TI Life issue, or contact me directly at my e-mail address … djlsuniverse@yahoo.com.